The spring growth flush

I’ve been getting a lot of questions about the turf GvX. And I’ve been checking my calculations to make sure I’ve got a somewhat standardized method to get the GvX number for any day.

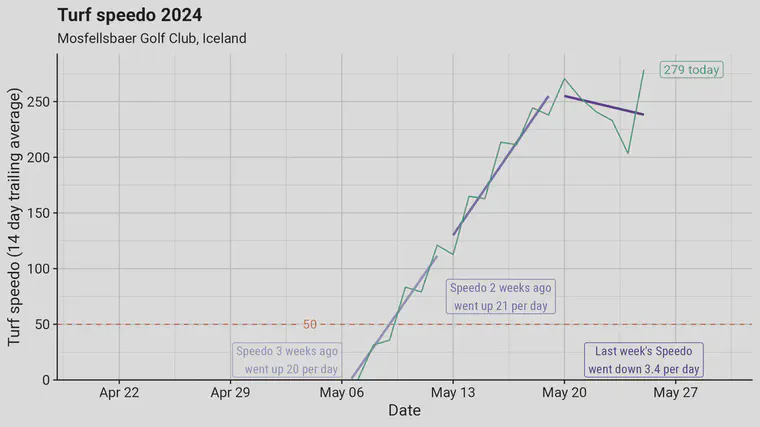
The chart above shows the grass jumping out of the gate to start the spring. Mosfellsbaer Golf Club is near Reykjavik. Bjarni Hannesson, the course manager, kindly shared the clipping volume and temperature data with me. There have been three days this year with an average air temperature above 10 °C (50 °F). The grass on the putting surfaces at Mosfellsbaer Golf Club, however, is growing at a rapid rate considering those rather cool temperatures: the turf GvX is currently at 279.

It is normal, when grass comes out of winter dormancy, for there to be a GvX above 100 in the spring. Have the greens at Mosfellsbaer already reached the peak of their spring growth flush? Maybe. After the GvX increased at a rate of 20 per day for the first two weeks of mowing, that trend stopped over the past week.

How I’m calculating this

Here’s how I’m getting the GvX (growth ratio) number.

I calculate two values for each day.

One is the 14 day average of clipping volume. I calculate this by taking the total clipvol for today plus the previous thirteen days, and I divide that by 14.

The second value I calculate is the 14 day average of growth potential (GP). I’ve got the average daily air temperature for today and for each of the previous thirteen days. From those I use the GP utility function to convert temperature to GP, and I then calculate the 14 day average for each day.

Then I calculate the GvX for each day as:

$$ \frac{ClipVol_{14}}{20(GP_{14})} $$

Where $ClipVol_{14}$ is the 14 day average clipvol, the units for clipvol are equivalent to mL/m² or the equivalent L/1000 m², $GP_{14}$ is the 14 day average GP on a scale of 0 to 1, and 20 is a constant.

The Chaotic Turf GvX

Why the calculations with an average over 14 days? Well, I’m trying to get an idea of how much the grass is growing given the recent weather conditions. I’ve done this with 30 day averages, but I realized I wasn’t so concerned with how the grass was growing in relation to the weather 28 days ago. I’ve done this with 7 day averages too. I thought that sometimes there aren’t enough mows in a week, or the weather changes too violently in one week, and I liked having that little bit of extra smoothing that comes from including data from the previous week. So a couple years ago I began calculating the GvX for most situations using the 14 day average. I’m not saying this is the only way to do it, but this is how I’m making the calculation right now.

Here’s how I’m not doing it.

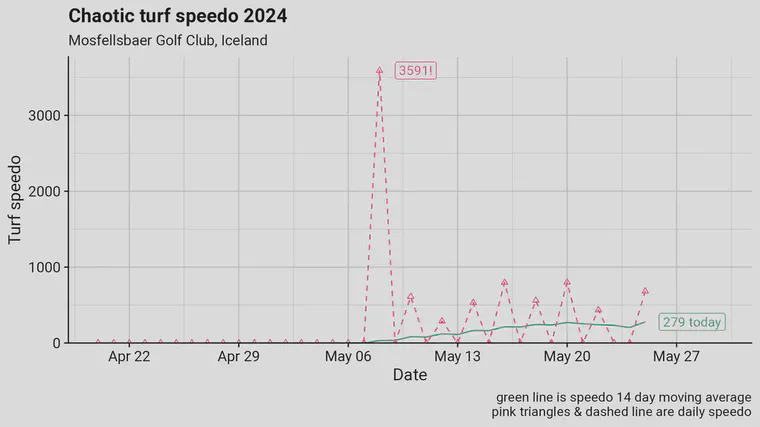
I’m not calculating the GvX every day based on that day’s clipvol only and on that day’s temperature only. If I did that, days without a mow would have a GvX of 0. But the grass is actually growing, and it is experiencing weather. It just didn’t happen to get mown on a day, and the GvX drops to 0? That’s not so informative. Or if I mowed a lot of grass on a particularly cold day, that could be a massive GvX.

Grass gets mown in starts and stops. But the GvX should be continuous. In order to have a continuous GvX during the mowing season, one needs to smooth the data at least as far as the duration between mows.